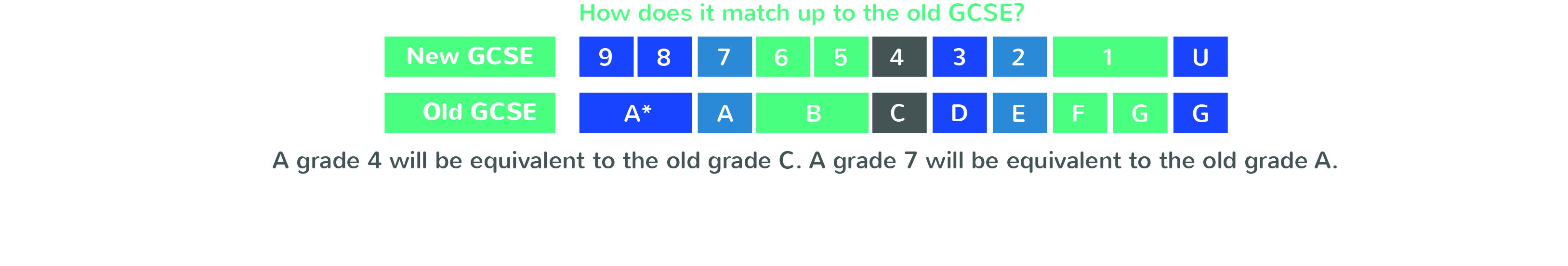
In 2017, the new GCSE papers were introduced, with a new grade format consisting of numbers: 9-1.

Below, you can find past papers and specimen papers for the Edexcel and AQA exam boards.

Register with 10ticks today and download our 10ticks GCSE revision cards, absolutely free!

Here you can find the past papers and specimen papers for the exam boards; Edexcel and AQA

All GCSEs are now based on Grades 9 (the top grade) to 1. Grade 4 is the equivalent to a pass.

Foundation covers grades 5,4,3,2,1 (U) and Higher covers 9,8,7,6,5,4 (U).

A Grade 8 pupil can:

• Perform procedures accurately
• Interpret and communicate complex information accurately
• Make deductions, inferences and draw conclusion
• Construct substantial chains of reasoning, including convincing arguments and formal proofs
• Generate efficient strategies to solve complex mathematical and non-mathematical problems by translating them into a series of processes
• Make and use connections, which may not be immediately obvious, between different parts of mathematics
• Interpret results in the context of the given problem
• Critically evaluate methods, arguments, results and the assumption made



A Grade 5 pupil can:

• Perform routine single and multi-step procedures effectively by recalling, applying and interpreting notation, terminology, facts, definitions and formulae
• Interpret and communicate information effectively
• Make deductions, inferences and draw conclusions
• Construct chains of reasoning, including arguments
• Generate strategies to solve mathematical and non-mathematical problems by translating them into mathemathical processes, realising connections between different parts of mathematics
• Interpret results in the context of the given problem
• Evaluate methods and results



A Grade 2 pupil can:

• Recall and use notation, terminology, facts and definitions: perform routine procedures, including some multi-step procedures
• Interpret and communicate basic information; make deductions and use reasoning to obtain results.
• Solve problems by translating simple mathematical and non-mathmatical problems into mathematical processes
• Provide basic evaluation of methods or results
• Interpret results in the context of the given problem